NCERT Solutions class 10 maths chapter 7

Coordinate Geometry

NCERT Solutions class 10 maths chapter 7 Exercise 7.2

Question 1 Find the coordinates of the point which divides the join of (- 1, 7) and (4, – 3) in the ratio 2:3.

Answer

Let P(x, y) be the required point.
the section formula x=ss
and y=
So x₁ = -1 , x₂ =4 ,y₁=7 ,y₂= -3
and m₁ = 2 , m₂ =3
so
x = = (8-3)/5 = 5/5 =1
y = = (-6+21)/5 = 15/5 = 3
the point is (1, 3)

Question 2

. Find the coordinates of the points of trisection of the line segment joining (4, -1) and (-2, -3).

Answer

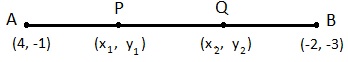
. Here Let assume P (x₁, y₁) and Q(x₂, y₂₂) are the points of trisection so AP = PQ = QB 1:2. here we know
x =
y =
x₁ =
= (-2+8)/3 = 6/3 = 2
y₁ =
= (-3-2)/3 = -5/3
Therefore: P (x₁, y₁) = P(2, -5/3) Point Q divides AB internally in the ratio 2:1. x₂ =
= (-4+4)/3 = 0
y₂ =
= (-6-1)/3 = -7/3
The coordinates of the point Q is (0, -7/3)

Question 3

To conduct Sports Day activities, in your rectangular shaped school ground ABCD, lines have been drawn with chalk powder at a distance of 1 m each. 100 flower pots have been placed at a distance of 1 m from each other along AD, as shown in the following figure. Niharika runs 1/4 th the distance AD on the 2nd line and posts a green flag. Preet runs 1/5th the distance AD on the eighth line and posts a red flag. What is the distance between both the flags? If Rashmi has to post a blue flag exactly halfway between the line segment joining the two flags, where should she post her flag?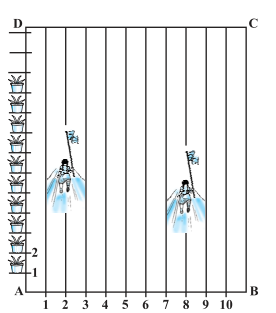

.

Answer

we observed that Niharika posted the green flag at 1/4th of the distance AD i.e., (1/4 ×100) m = 25m from the starting point of 2nd line. Therefore, the coordinates of this point are (2, 25).
Similarly, Preet posted red flag at 1/5 of the distance AD i.e., (1/5 ×100) m = 20m from the starting point of 8th line. Therefore, the coordinates of this point are (8, 20).
Distance between these flags
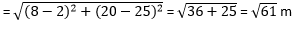
. The point at which Rashmi should post her blue flag is the mid- point of the line joining these points. Let say this point be B(x, y).
x = (2+8)/2 = 10/2 = 5
y = (20+25)/2 = 45/2
B( x, y) = (5,45/2)
Therefore, Rashmi should post her blue flag at 45/2 = 22.5m on 5th line.

Question 4

Find the ratio in which the line segment joining the points (-3, 10) and (6, – 8) is divided by (-1, 6).

Answer

Let assume the ratio in which the line segment joining ( -3, 10) and (6, -8) is divided by point ( -1, 6) be k :1.
So , -1 =

–k – 1 = 6k -3
7k = 2
k = 2/7
Therefore, the required ratio is 2:7.

Question 5

Find the ratio in which the line segment joining A (1, – 5) and B (- 4, 5) is divided by the x-axis. Also find the coordinates of the point of division.

Answer

Let the ratio in which the line segment joining A (1, – 5) and B ( – 4, 5) is divided by x-axis be k : 1.
Therefore, the coordinates of the point of division, say Q(x, y) is , .
We know that y-coordinate of any point on x-axis is 0.
Therefore, = 0
5k = 5
or k = 1
So, x-axis divides the line segment in the ratio 1:1.
the point of division:
Q (x, y) =, .
= , .= (-3/2 , 0)

Question 6

If (1, 2), (4, y), (x, 6) and (3, 5) are the vertices of a parallelogram taken in order, find x and y.

Answer

Let A,B,C and D be the vertex of a parallelogram : A(1,2), B(4,y), C(x,6) and D(3,5). and O is intersection point of diagonal AC and BD

.
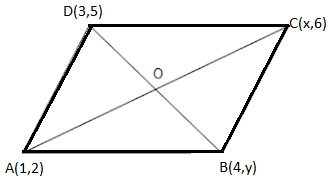
Since O is the midpoint of AC and BD .
Midpoint of AC
= ( (1+x)/2 , (2+6)/2 ) = ((1+x)/2 , 4)
Midpoint of BD
= ((4+3)/2 , (5+y)/2 ) = (7/2 , (5+y)/2)
Midpoint of AC and BD are same
(1+x)/2 = 7/2 and 4 = (5+y)/2
x + 1 = 7 and 5 + y = 8
x = 6 and y = 3.

Question 7

Find the coordinates of a point A, where AB is the diameter of circle whose centre is (2, – 3) and B is (1,4).

Answer

Let the coordinates of point A be (x, y).
Mid-point of AB is (2, – 3), which is the centre of the circle.
Coordinate of B = (1, 4)
(2, -3) =((x+1)/2 , (y+4)/2)
(x+1)/2 = 2 and (y+4)/2 = -3
x + 1 = 4 and y + 4 = -6
x = 3 and y = -10
The coordinates of A(3,-10).

Question 8

If A and B are (-2, -2) and (2, -4), respectively, find the coordinates of P such that AP = 3/7 AB and P lies on the line segment AB.

Answer

.
The coordinates of point A and B are (-2,-2) and (2,-4) respectively. Since AP = 3/7 AB
Therefore, AP: PB = 3:4
Point P divides the line segment AB in the ratio 3:4.
cordinate of P = ,
= ,
= ,

Question 9

Find the coordinates of the points which divide the line segment joining A (- 2, 2) and B (2, 8) into four equal parts.

Answer

.

From the figure, it can be observed that points X, Y, Z are dividing the line segment in a ratio 1:3, 1:1, 3:1 respectively.
Cordinate of X = ,
= - 1 ,
cordinate of Y = ,
= (0 ,5 )
Cordinate of Z = ,
= - 1 ,

Question 10

Find the area of a rhombus if its vertices are (3, 0), (4, 5), (-1, 4) and (-2,-1) taken in order.
[Hint: Area of a rhombus = 1/2(product of its diagonals)

Answer

Let A(3, 0), B (4, 5), C( – 1, 4) and D ( – 2, – 1) are the vertices of a rhombus ABCD.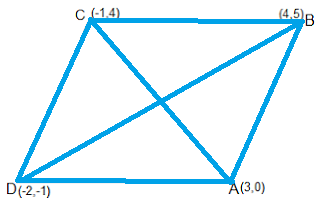

.

.