NCERT Solutions for class 10 maths chapter 2

Polynomials

NCERT Solutions for class 10 maths chapter 2 Exercise 2.3

Question 1

Divide the polynomial p(x) by the polynomial g(x) and find the quotient and remainder in each of the following:

(i)p(x) = x³-3x²+5x–3 , g(x) = x²–2

(ii) p(x) = x⁴-3x²+4x+5 , g(x) = x²+1-x

(iii) p(x) =x⁴–5x+6, g(x) = 2–x²

Answer

(i)p(x) = x³-3x²+5x–3 , g(x) = x²–2

Here

Dividend = p(x) = x³-3x²+5x–3

Divisor = g(x) = x²– 2

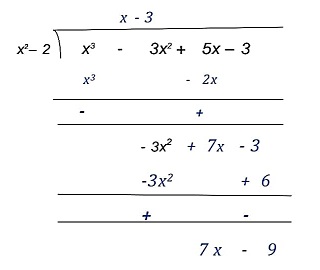
we get,

Quotient = x–3

Remainder = 7x–9

(ii) p(x) = x⁴-3x²+4x+5 , g(x) = x²+1-x

Answer

Given,

Dividend = p(x) = x⁴ – 3x² + 4x +5

Divisor = g(x) = x² +1-x

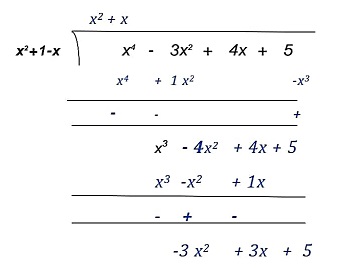
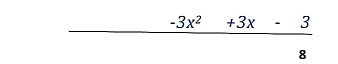
we get,

Quotient = x² + x–3

Remainder = 8

(iii) p(x) =x⁴–5x+6, g(x) = 2–x²

Answer

Given,

Dividend = p(x) =x⁴ – 5x + 6 = x⁴ +0x²–5x+6

Divisor = g(x) = 2–x² = –x²+2

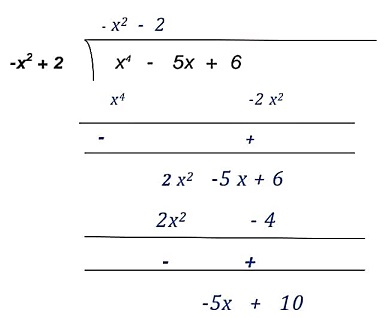
Therefore, upon division we get,

Quotient = -x²-2

Remainder = -5x + 10

Question 2

Check whether the first polynomial is a factor of the second polynomial by dividing the second polynomial by the first polynomial:

(i) t²-3, 2t⁴ +3t³-2t²-9t-12

(ii)x²+3x+1 , 3x⁴+5x³-7x²+2x+2

(iii) x³-3x+1, x⁵-4x³+x²+3x+1

Answer

(i) t²-3, 2t⁴ +3t³-2t²-9t-12

Given,

First polynomial = t²-3

Second polynomial = 2t⁴ +3t³-2t² -9t-12

As remainder is 0. so we can say that, t²-3 is a factor of 2t²+3t+4.

(ii)x²+3x+1 , 3x⁴+5x³-7x²+2x+2

Answer

Given,

First polynomial = x²+3x+1

Second polynomial = 3x⁴+5x³-7x²+2x+2

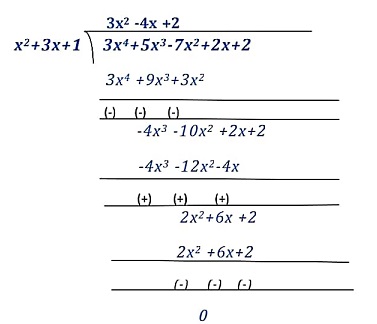
As remainder is 0. so, we can say that, x² + 3x + 1 is a factor of 3x⁴+5x³-7x²+2x+2.

(iii) x³-3x+1, x⁵-4x³+x²+3x+1

Answer

Given,

First polynomial = x³-3x+1

Second polynomial = x⁵-4x³+x²+3x+1

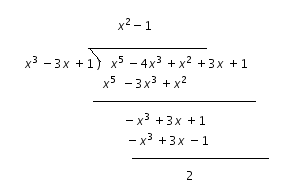
As remainder is not equal to 0. So, we say that, x³-3x+1 is not a factor of x⁵-4x³+x²+3x+1 .

Question 3

Obtain all other zeroes of 3x⁴+6x³-2x²-10x-5, if two of its zeroes are √(5/3) and – √(5/3).

Answer

√(5/3) and – √(5/3) are zeroes of f(x).
so

(x –√(5/3) (x+√(5/3) = x²-(5/3) = 0

(3x²−5)=0, is a factor of given polynomial f(x).

Now,

Therefore, 3x⁴ +6x³ −2x² −10x–5 = (3x² –5)(x²+2x+1)

On further factorizing (x²+2x+1) we get,

x²+2x+1 = x²+x+x+1 = 0

x(x+1)+1(x+1) = 0

(x+1)(x+1) = 0

So, its zeroes are given by: x= −1 and x = −1.

Therefore, all four zeroes of polynomial equation are:

√(5/3),- √(5/3) , −1 and −1.

Question 4

On dividing x³-3x²+x+2 by a polynomial g(x), the quotient and remainder were x–2 and –2x+4, respectively. Find g(x).

Answer

Given,

Dividend, p(x) = x³-3x²+x+2

Quotient = x-2

Remainder = –2x+4

we know,

Dividend = Divisor × Quotient + Remainder

∴ x³-3x²+x+2 = g(x)×(x-2) + (-2x+4)

x³-3x²+x+2-(-2x+4) = g(x)×(x-2)

Therefore, g(x) × (x-2) = x³-3x²+x-2

g(x) =

Therefore, g(x) = (x²–x+1)

Question 5

Give examples of polynomials p(x), g(x), q(x) and r(x), which satisfy the division algorithm and

(i) deg p(x) = deg q(x)

(ii) deg q(x) = deg r(x)

(iii) deg r(x) = 0

Answer

(i) deg p(x) = deg q(x)

We know
Dividend = Divisor × Quotient + Remainder

∴ p(x) = g(x)×q(x)+r(x)

Where r(x) = 0 or degree of r(x)< degree of g(x).

(i) deg p(x) = deg q(x)

Answer

Degree of dividend is equal to degree of quotient, only when the divisor is a constant term.

Let us assume an example, 6x²+6x+6 is a polynomial to be divided by 3.

p(x)= 6x²+6x+6
g(x)= 3

So, (6x²+6x+6)/3 = 2x²+2x+2 = q(x)

so we can see, the degree of p(x) and q(x) is equal.

Hence, division algorithm is satisfied here.

(ii) deg q(x) = deg r(x)

Answer

Let us p(x)=x⁴+x
g(x)=x³.

So, (x⁴+x)/x³ = x = q(x)

Also, remainder, r(x) = x

we can see, the degree of q(x) and g(x) is equal .

Hence, division algorithm is satisfied here.

(iii) deg r(x) = 0

Answer

Let us take an example, p(x) = x³+1 is a polynomial to be divided by g(x)=x.

So,( x³+1)/x= x=q(x)

And r(x)=2

Clearly, the degree of remainder r(x) is 0.

Hence, division algorithm is satisfied here.