NCERT Solutions class 10 maths chapter 13

Surface Areas and Volumes

NCERT Solutions class 10 maths chapter 13 Exercise 13.2

Exercise 13.1 Exercise 13.3 Exercise 13.4 Exercise 13.5

Question 1

. A solid is in the shape of a cone standing on a hemisphere with both their radii being equal to 1 cm and the height of the cone is equal to its radius. Find the volume of the solid in terms of π.

Answer 1

Here r = 1 cm and h = 1 cm.

Now, Volume of solid = Volume of conical part + Volume of hemispherical part

We know taht volume of cone = (1/3)πr²h

And,

The volume of hemisphere = (2/3)πr³

So, volume of solid will be

(1/3)π(1)²[1 + 2(1)]cm³ =(1/3)π x 1 x [3]cm³

= π cm³

Question 2

. Rachel, an engineering student, was asked to make a model shaped like a cylinder with two cones attached at its two ends by using a thin aluminum sheet. The diameter of the model is 3 cm and its length is 12 cm. If each cone has a height of 2 cm, find the volume of air contained in the model that Rachel made. (Assume the outer and inner dimensions of the model to be nearly the same.)

Answer 2

Given,

Height of cylinder = 12–4 = 8 cm

Radius = 1.5 cm

Height of cone = 2 cm

Now, the total volume of the air contained will be = Volume of cylinder+2×(Volume of cone)

∴ Total volume = πr²h+[2×(⅓ πr²h )]

= 18 π+2(1.5 π)

= 66 cm³.

Question 3

A Gulab jamun contains sugar syrup up to about 30% of its volume. Find approximately how much syrup would be found in 45 Gulab jamuns, each shaped like a cylinder with two hemispherical ends with length 5 cm and diameter 2.8 cm (see figure).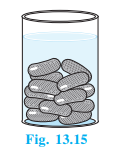

Answer 3

So, the total height of a gulab jamun = 5 cm.

Diameter = 2.8 cm

So, radius = 1.4 cm

∴ The height of the cylindrical part = 5 cm–(1.4+1.4) cm

=2.2 cm

Now, volume of One Gulab Jamun = Volume of Cylinder + Volume of two hemispheres

= πr²h+(4/3)πr³

= 4.312π+(10.976/3) π

= 25.05 cm³

volume of sugar syrup = 30% of total volume

So, volume of sugar syrup in 45 gulab jamuns = 45×30%(25.05 cm³)

= 45×7.515 = 338.184 cm³

Question 4

A pen stand made of wood is in the shape of a cuboid with four conical depressions to hold pens. The dimensions of the cuboid are 15 cm by 10 cm by 3.5 cm. The radius of each of the depressions is 0.5 cm and the depth is 1.4 cm. Find the volume of wood in the entire stand (see Fig.).

Answer

Volume of cuboid = length x width x height

length = 15cm
width = 10cm
height =3.5cm

So, the volume of the cuboid = 15x10x3.5 = 525 cm³

Given, radius r = 0.5 cm and height h = 1.4 cm

Here, volume of conical depressions

= (1/3)πr²h

∴ Volume of 4 cones = 4x(1/3)πr²h

= 1.46 cm²

Now, volume of wood = Volume of cuboid – 4 x volume of cone

= 525-1.46 = 523.54 cm²

Question 5

A vessel is in the form of an inverted cone. Its height is 8 cm and the radius of its top, which is open, is 5 cm. It is filled with water up to the brim. When lead shots, each of which is a sphere of radius 0.5 cm are dropped into the vessel, one-fourth of the water flows out. Find the number of lead shots dropped in the vessel.

Answer 5

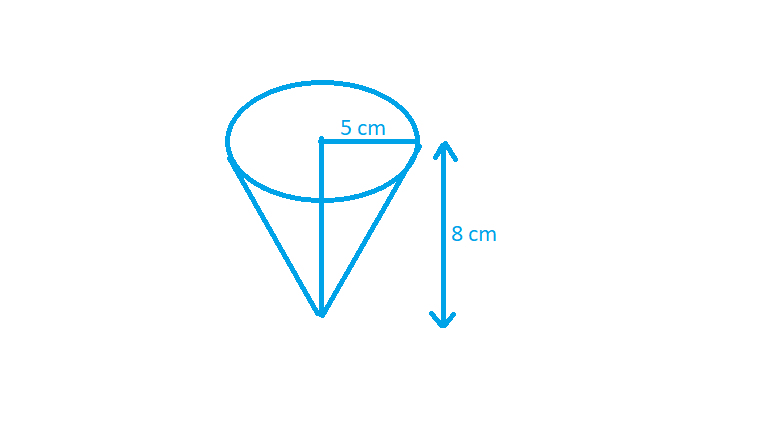
For the cone,

Radius = 5 cm,

Height = 8 cm

Also,

Radius of sphere = 0.5 cm

volume of water in vessel

=(1/3) x π x r² x h

(1/3) x (22/7) x 5 x 5 x 8
=(4400/21)cm³

Volume of lead shot

= (4/3)πr³

= (1/6) x (22 /7) = (11/21)cm³

let n is the number of lead shots dropped in the vessel

So,
n x volume of water in vessel = (1/4) x volume of water in vessel

n x (11/21) = (4400/21 ) x (1/4)
= n x 11 =1100
n=100

So the number of lead shots =100

Question 6

A solid iron pole consists of a cylinder of height 220 cm and base diameter 24 cm, which is surmounted by another cylinder of height 60 cm and radius 8 cm. Find the mass of the pole, given that 1 cm³ of iron has approximately 8 g mass.

Answer 6

Given, the height of the big cylinder H = 220 cm

Radius of the base R = 24/12 = 12 cm

Now, the height of smaller cylinder (h) = 60 cm

Radius of the base (r) = 8 cm

So, the volume of the smaller cylinder = πr²h

= π(8)2×60 cm³

= 12068.5 cm³

∴ Volume of iron pole = Volume of the big cylinder+ Volume of the small cylinder

= 99565.8 + 12068.5

=111634.5 cm³

We know,

Mass = Density x volume

So, mass of the pole = 8×111634.5

= 893.26 Kg

Question 7

A solid consisting of a right circular cone of height 120 cm and radius 60 cm standing on a hemisphere of radius 60 cm is placed upright in a right circular cylinder full of water such that it touches the bottom. Find the volume of water left in the cylinder, if the radius of the cylinder is 60 cm and its height is 180 cm.

Answer 7

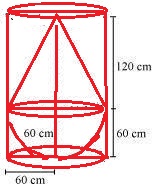
Given,

Radius of cone r₁ = 0.6 m,

Height of cone h₁= 1.2 m

Radius of cylinder r = 0.6 m

Height of cylinder h= 1.8 m

Radius of hemisphere r₂= 0.6 m

Now,

Volume of solid = volume of cone + volume of the hemisphere

=(1/3)x π x r²h₁ + (2/3) x π x r₂³

= (1/3) x (22/7) x (0.6)² x (1.2) + (2/3) x (22/7) x (0.6)³

= (22/21) x (0.6)²[ 1.2 + 2 x 0.6]

= (22/21) x 0.36 (1.2 + 1.2)

= 6.336/7 m³

volume of water left in cylinder = volume of water filled in circular cylinder - volume of solid

(14.256/7 ) - (6.336/7) = 1.131 m³

Question 8

A spherical glass vessel has a cylindrical neck 8 cm long, 2 cm in diameter; the diameter of the spherical part is 8.5 cm. By measuring the amount of water it holds, a child finds its volume to be 345 cm³. Check whether she is correct, taking the above as the inside measurements, and π = 3.14.